Задача 19
. Найти производную второго порядка 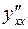 от функции, заданной параметрически.
19.1.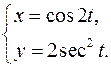 
x'= -2sin2t= -4sintcost
y'= 4sint/cos3
t
y''xx
= 4sint
= -1 _
16sin2
tcos5
t 4sintcos5
t
19.2.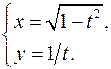 
x'= -t/√(1-t2
)
y'= -1/t2
y''xx
= (1-t2
)2
t4
19.3.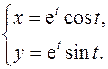 
x'= et
cost-et
sint= et
(cost-sint)
y'= et
sint+et
cost= et
(sint+cost)
y''xx
= et
(sint+cost)
= sint+cost
e2t
(cost-sint)2
et
(cost-sint)2
19.4.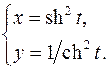 
x'= 2shtcht
y'= -2sht/ch3
t
y''xx
= -2
sht
= -1_
4shtch4
t 2ch4
t
19.5.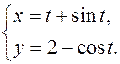 
x'= 1+cost
y'= sint
y''xx
= sint/(1+cost)2
19.6.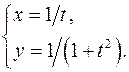 
x'= -1/t2
y'= -2t/(1+t2
)2
y''xx
= -2t3
_
(1+t2
)2
19.7.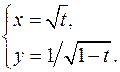 
x'= 1/2√t
y'= 1/√(1-t)3
y''xx
= 4
t _
√(1-t)3
19.8.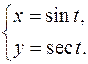 
x'= cost
y'= sint/cos2
t
y''xx
= sint/cos4
t
19.9.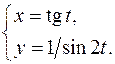 
x'= 1/cos2
t
y'= -2cos2t/sin2
2t
y''xx
= -2cos2tcos4
t
sin2
2t
19.10.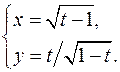 
x'= 1/2√(t-1)
y'= (2-t)/(1-t)3/2
y''xx
= 4(
t
-1)(2-
t
)
= 2
t
-8
(1-t)3/2
√(1-t)
19.11.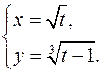 
x'= 1/2√t
y'= 1/3
√(t-1)2
y''xx
= 4t/3
√(t-1)2
19.12.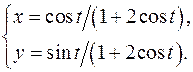 
x'= -sint/(1+2cost)2
y'= (cost+2)/(1+2cost)2
y''xx
= (
cost+2)(1+2cost)4
= (
cost+2)(1+2cost)2
sin2
t(1+2cost)2
sin2
t
19.13.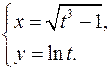 
x'= 3t2
/ 2√(t3
-1)
y'= 1/t
y''xx
= 2
(
t
3
-1)
3t5
19.14.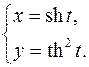 
x'= cht
y'= 2tht/ch2
t
y''xx
= 2tht/ch4
t
19.15.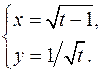 
x'= 1/2√(t-1)
y'= -1/2√t3
y''xx
= -
2t
+
2
√t3
19.16.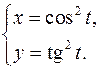 
x'= -2cost sint
y'= 2sint/cos3
t
y''xx
= 2sint
= 1/2cos4
t
4cos4
tsint
19.17.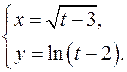 
x'= 1/2√(t-3)
y'= 1/(t-2)
y''xx
= 4(t-3)/(t-2)
19.18.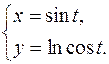 
x'= cost
y'= -sint/cost
y''xx
= -sint/cos3
t
19.19.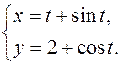 
x'= 1+cost
y'= -sint
y''xx
= -sint/(1+cost)2
19.20.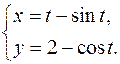 
x'= 1-cost
y'= sint
y''xx
= sint/(1-cost)2
19.21.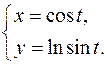 
x'= -sint
y'= cost/sint
y''xx
= -cost/sin3
t
19.22.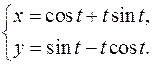 
x'= -sint+sint+tcost= tcost
y'= cost-cost+tsint= tsint
y''xx
= sint/cos2
t
19.23.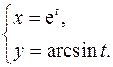 
x'= et
y'= 1/√(1-t2
)
y''xx
= et
/√(1-t2
)
19.24.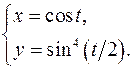 
x'= -sint
y'= 2sin3(t/2)cos(t/2)
y''xx
= -2sin3
(t/2)cos(t/2)/sint= -sin2
(t/2)
19.25.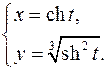 
x'= sht
y'= 2cht/33
√sht
y''xx
= 2cht/33
√sh4
t
19.26.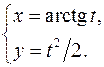 
x'= 1/(1+t2
)
y'= t
y''xx
= t(1+t2
)2
19.27.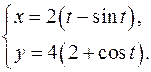 
x'= 2-2cost
y'= -4sint
y''xx
= -2sint/(1-cost)
19.28.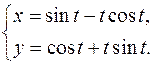 
x'= cost-cost+tsint= tsint
y'= -sint+sint+tcost= tcost
y''xx
= cost/sin2
t
19.29.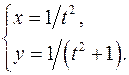 
x'= -2/t3
y'= -2t/(t2
+1)2
y''xx
= -t7
/2(t2
+1)2
19.30.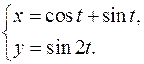 
x'= cost-sint
y'= 2cos2t
y''xx
= 2cos2t/( cost-sint)= 2cost+2sint
19.31.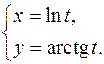 
x'= 1/t
y'= 1/(1+t2
)
y''xx
= t2
/(1+t2
)
|
